WoG 2021 Talk 4.5: Mariarlaura Noce - Ramification structures for quotients of the Grigorchuk groups

Speaker: Marialaura Noce (U. Göttingen)

Title: Ramification structures for quotients of the Grigorchuk groups
Abstract: Groups of automorphisms of regular rooted trees have been studied for years as an important source of groups with interesting properties. For example, the Grigorchuk groups provide a family of groups with intermediate word growth and the torsion Grigorchuk groups constitute a counterexample to the General Burnside Problem. For these groups there is a natural family of normal subgroups of finite index, which are the level stabilizers. The goal of this talk is to show that the quotients by such subgroups admit a ramification structure. Roughly speaking, groups of surfaces isogenous to a higher product of curves are characterised by the existence of a ramification structure. Recall that an algebraic surface $S$ is isogenous to a higher product of curves if it is isomorphic to $(C_1 \times C_2)/G$, where $C_1$ and $C_2$ are curves of genus at least $2$, and $G$ is a finite group acting freely on $C_1 \times C_2$.

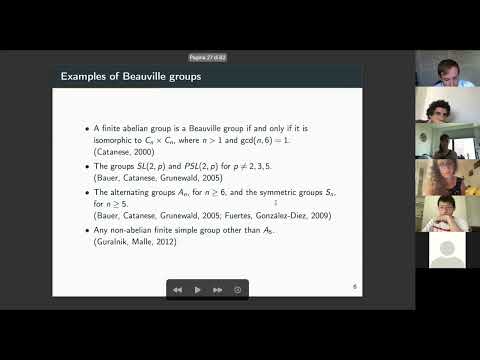
In this talk, we first introduce the Grigorchuk groups and then we show that their quotients admit ramification structures, providing the first explicit infinite family of $3$-generated finite $2$-groups with ramification structures that are not Beauville. This is joint work with A. Thillaisundaram.

MariarlauraRamificationstructures